Miscellaneous

Trigonometry Formulas

Determining Sides and Angles of a Right Triangle

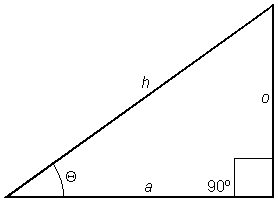
Right triangle with its sides labeled h, a, and o and the interior angle opposite of side o labeled theta        a2 + o2 = h2 (Pythagorean theorem)
 
sin theta = o   csc theta = 1  =  h
 h  sin theta  o 
 
cos theta = a   sec theta = 1  =  h
 h  cos theta  a 
 
tan theta = o  =  sin theta        cot theta = 1  =  a
 a  cos theta tan theta  o 


Determining Sides and Angles of Any Triangle

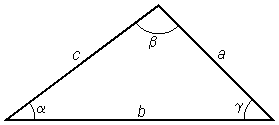Any triangle with the sides labeled a, b, and c and the opposite interior angles labeled alpha, beta, and gamma        sin alpha  =  sin beta  =  sin gamma    (Law of Sines)
a b c
 
c2 = a2 + b2 - 2ab cos gamma    (Law of Cosines)

Known Sides and Angles     Example
All three sides (SSS)
a, b, and c		 cos alpha = b2 + c2 - a2     
2bc
Two sides and the angle
included between them (SAS)
b, alpha, and c		 a2 = b2 + c2 - 2bc cos alpha
Two sides and an angle not
included between them (SSA)
a, b, and alpha		 sin beta = b sin alpha     
a
One side and two angles (SAA)
a, alpha, and beta		 b = a sin beta     
sin alpha


Correcting Rotated Graph Axes

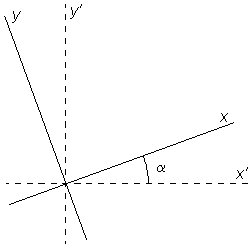X and Y axes rotated about the origin by alpha degrees, X' and Y' being the corrected axes.        alpha = tan-1(slope of x)

x' = x cos alpha + y sin alpha

y' = -x sin alpha + y cos alpha

[  Index  |  Technical Notes Page  ]

This page is for INFORMATIONAL PURPOSES ONLY.

Page author: Dawn Rorvik (rorvikd@evergreen.edu)
Last modified: 03/28/1999